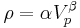Gardner's relation

Gardner's relation, or Gardner's equation, named after G. H. F. Gardner and L. W. Gardner, is an empirically derived equation that relates seismic P-wave velocity to the bulk density of the lithology in which the wave travels. The equation reads:

$\rho = \alpha V_p^{\beta}$

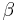where $\rho$ is bulk density given in g/cc, $V_p$ is P-wave velocity given in ft/s, and $\alpha$ and $\beta$ are empirically derived constants that depend on the geology. Gardner et al. proposed that one can obtain a good fit by taking $\alpha = 0.23$ and $\beta = 0.25$ .^[1] Assuming this, the equation is reduced to:

$\rho = 0.23 V_p^{0.25}.$

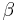This equation is very popular in petroleum exploration because it can provide information about the lithology from interval velocities obtained from seismic data. The constants $\alpha$ and $\beta$ are usually calibrated from sonic and density well log information but in the absence of these, Gardner's constants are a good approximation.

References

^ Gardner, G.H.F.; Gardner L.W. & Gregory A.R. (1974). "Formation velocity and density -- the diagnostic basics for stratigraphic traps". Geophysics 39: 770–780. http://www.ipt.ntnu.no/pyrex/stash/GPY00770.pdf.